GMAT Day 8

Ok, so as it happens the last 2 days I have had too much work and was not able to do much (yesterday post is actually from 2 days back I think? I just forgot to post it and got saved as a draft. While I am between meetings I use prep4gmat app to learn verbal reasoning and so far I am really enjoying it and it is helping me quite a lot I would say. I definitely recomend that app .

Geometry

At last… we get into… Geometry! For some reason I am not looking  forward… so let’s re-wire the mind. Let’s enjoy it, let’s have fun, let’s be great at geometry, let’s go.

Angles

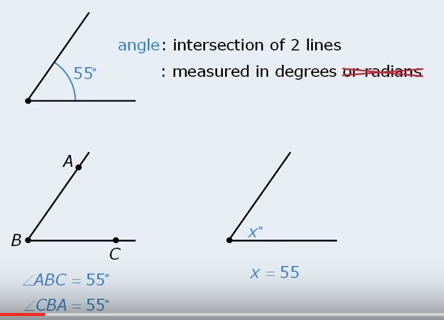
Ok so went through the basics of angles on a line. Important learned the notation <ABC , this means the angle is coming from B.

Properties of lines easy no need to write .

Triangles

• All angles add to 180 degree
• The longest side is opposite to the largest angle
• The shorter side is opposite the smallest angle
• Those two can be summarized as if a<b<c then A<B<C. This is important to really understand… so if one asks is angle x > than y is the same as asking is the opposite side to x larger than opposite side to y?
• The sum of the lengths of any two sides of a triangle must be greater than the third side. For example if sides are 3 and 7, what lenghts are possible for the third side? a positive number <10 and > 4 is the answer. (So any 3rd side has to be greater than abs(difference other two sides) and less than sum of other two sides.

Consider this question

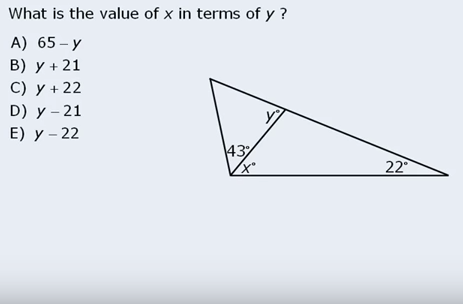
This I am happy to solve correctly and quickly in < 1min. i knwo it’s easy but still… happy its working. Important to celebrate small victories XD!

Assumptions

On GMAT all lines that seems straight are straight. However this doesn’t mean u can assume angles. So if it looks perpendicular doesn’t mean its perpendicular for example.

Can’t assume 2 lines are paralel because they look like.

Angles that appear on diagram are bigger than 0 degrees.

Triangles isosceles  / equilateral

2 sides are equal and the 2 angles opposite are equal too of course.  If we know 1 angle, we can always know the other 2 angles.

Equilateral has 3 equal sides and 3 angles same, so 60 degree each.

Area of triangle

a = bh/2

Area of equilateral triangle = (Root(3)*side^2)/4 – I don’t like to memorize formulas but well, I guess I will have to.

Rule: Altitudes of equilateral and isosceles triangles always bisect the base

Right and special triangles properties

Hypotenuse is longest side (as it’s the one oposite of the 90 degree angle which will always be the largest angle in a right triangle).

Pythagoras theorem. Ina  right triangle  h^2 = a^2 + b^2

Pythagorean triple: A set of 3 integers that can be the sides of a right triangle. Here seems good to memorize these ones: 3-4-5 , 5-12-13, 8-15-17, 7-24-25. Their multiples are also pythagorean triples. This can only be used if one has 2 CORRESPONDING sides. Just one side or 2 non correspomding sides doesn’t cut it.

Consider this question

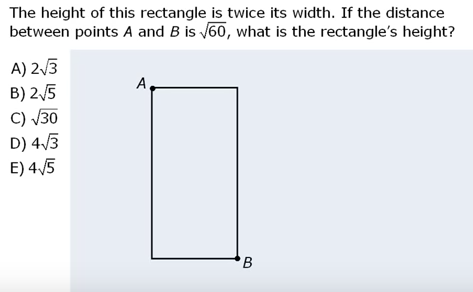
Now it is easy however, remember it asks the height. I calculated side right, and made a mistake as it asks height not width. I MUST always try as best as possible to re-write question to make sure I am answering what they are asking.

A special triangle is the 45-45-90 or 90-60-30 as below:

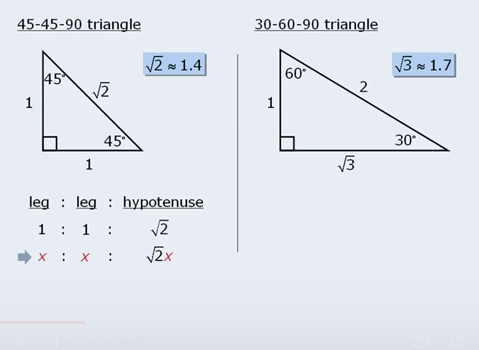
This is important because it is the only way to for example find one side of a special triangle… if I only have 1 angles besides the right one, and one side.

Thesespecial triangles hide between squares and also equilateral triangles. Watch out for them

Here an example of a tricky question that basically can only be answered if you know those rules:

Now, I actually got confused, first because angle ABC I confused with angle ABD. Didn’t look careful enough on the diagram. Then later once I fixed that, I again made another error concluding that an isosceles right triangle has angles 90 55 55 which obviously doesnt make sense, it is 90 45 45, which allows us to use the properties, also this shows that the bottom triangle is a 90-60-30 one, which again allows us to easily solve for X.

 

Similar triangles have 3 angles in common

So one is the same triangle magnified.

Rule: With similar triangles, the ratio of any pair of corresponding sides is the same

Quadrilateral

It is a poligon with 4 sides. Square, Parallellogram, rectangle, trapezoid rombus

Rule 1: Angles add to 360 degrees

Parallelogram has 2 pairs of parallel sides (opposite angles equal, sides opposite equal). Rectangle is a type of parallelogram. Rombus also. Opposite angles equal and opp sides equal… actually in rombus 4 equal sides too.

Trapezoid is not paralelogram, but has 2 parallel.

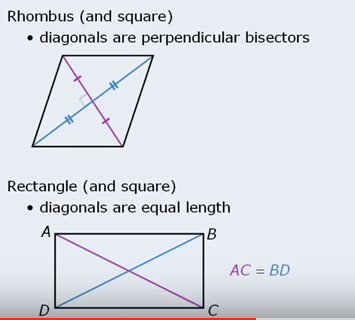
Other important rules. In rombus, diagonals are perpendicular bisectors. And in rectangle and square diagonas have equal length.

Area of quadrilateral is b*h

Area of trapezoid is ((base1 + base 2)/2) * height (So basically the average of two parallel sides multiplied by height).

Area of rombus can also be : (diagonal1 * diagonal2) / 2

Polygon

Closed figure formed by 3 or more lines.

All interior angles inside are less than 180 degrees. We have already seen triangle and quadrilateral.

 

Important rule 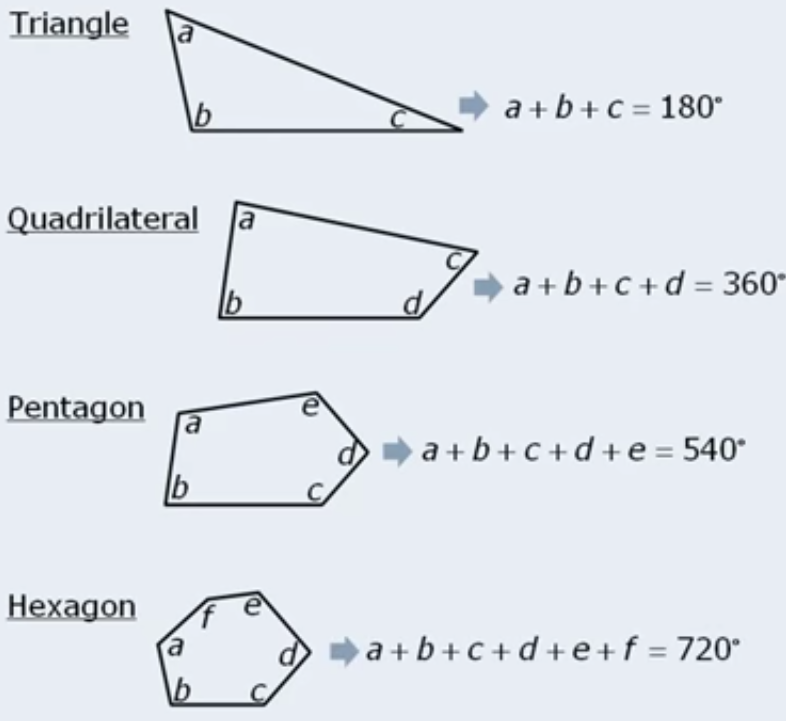to find sum of angles inside a polygon.

Angles inside polygon = 180*(N-2)

One can see why this is the case if draw line from one point to all other intersections. You have (N-2) number of triangles, and polygon must have all those angles inside hence the formula.

A “REGULAR” polygon means it has equal sides and equal angles.

Circles

• Points all at a given distance from the circle (Radius)
• A line from a point of the circle to another is called a CHORD. The LONGEST POSSIBLE CHORD on a circle is the Diameter.
• Arc is the perimeter between 2 points (minor arc).
• Circumference is 2*(PI)*radius.
• Area is PI*r^2
• Length of an arch of angle X is X/360 of a circumference
• Area of a sector of angle X is X/360 of a circumference

Circles properties

INSCRIBED ANGLE (is the angle opposite to a chord, when we have a triangle formed by a chord and one other point in the circle)

RULE 1: Inscribed angles at the same side of the chord and holding the same chord/arc are always equal

RULE2: Inscribed angles holding chords of equal length are equal

RULE 3: Inscribed angles holding de diameter is a right angle

An angle is a central angle when its vertex is at the center of the circle. Holds chord AB

RULE 4: If we have a central angle holding chord AB and we have an inscribed angled holding chord AB, then the CENTRAL ANGLES IS 2X the INSCRIBED ANGLE

RULE 5: I didn’t know this rule but basically inscribed angle creates a chord yes, but also an intercepted arc. The rule is that the intercepted arc is twice the measure of the inscribed angle. So the inscribed angle is half the intersected measure. 

A line that touches cercle side, is TANGENT to the circle.  A line from the center to the tangential line is 90 degree.

Volume and surface area

Volume of cilinder is Area of base * height .

Surface area of a rectangle with 6 faces. 12 edges and 8 vertices. Just add areas of 3 faces.

Surface area of a cylinder is the sum of the 2 circles + the side area which is perimeter of circle * the height.

Data sufficiency

To find one length we need at least one other length. Usually sufficient if forces the shape to having one length.

Three hours went by. I finished this section, now there are 14 questions. Will try finish them now.

Consider this:

Geometry questions can be damn hard. Hmm…

Big conclusions

Geometry is still a big weakness for me. I managed to answer most questions but its taking me way too long to answer them so it is totally insufficient. Need to practice this a lot after doing the general revision.

Geometry can be pretty tricky because there are so many relations one needs to take into account, formulas also… and you need to apply everything very quickly.

Gonna be tough guys to get this all right with the few time I have… but I will try!